14 (+3) Parameters for 3D Surface Roughness
 

This page includes fourteen parameters based on the project BRC No: 3374/1/0/170/90/2 and three new “volume” family parameters. 
 

AMPLITUDE PARAMETERS

Four parameters are used for characterizing the amplitude property of surfaces.  They are classified into four categories, i.e. (i) dispersion, (ii) extreme, (iii) asymmetry of the height distribution and (iv) sharpness of the height distribution.

(1). Root-Mean-Square Deviation of the Surface Sq
This is a dispersion parameter defined as the root mean square value of the surface departures within the sampling area.

                                     ( 1 )

where, M is a number of points of per profile, N is the number of profile.  Sq is a very general and widely used parameter.  In statistics, it is the sample standard deviation.

(2) Ten Point Height of the Surface Sz
This is an extreme parameter defined as the average value of the absolute heights of the five highest peaks and the depths of the five deepest pits or valleys within the sampling area. 

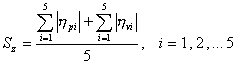                    ( 2 )

where  and  are the five highest surface summits and lowest surface valleys respectively, which rely on eight nearest neighbour summits. 

(3) Skewness of Topography Height Distribution Ssk
This is the measure of asymmetry of surface deviations about the mean plane.

                             ( 3 )

This parameter can effectively be used to describe the shape of the topography height distribution.  For a Gaussian surface which has a symmetrical shape for the surface height distribution, the skewness is zero.  For an asymmetric distribution of surface heights, the skewness may be negative if the distribution has a longer tail at the lower side of the mean plane or positive if the distribution has a longer tail at the upper side of the mean plane. This parameter can give some indication of the existence of "spiky" features. 

(4) Kurtosis of Topography Height Distribution Sku
This is a measure of the peakedness or sharpness of the surface height distribution.

                                     ( 4 )

This parameter characterizes the spread of the height distribution.  A Gaussian surface has a kurtosis value of 3.  A centrally distributed surface has a kurtosis value larger than 3 whereas the kurtosis of a well spread distribution is smaller than 3.  By a combination of the skewness and the kurtosis, it may be possible to identify surfaces which have a relatively flat top and deep valleys.
 

SPATIAL PARAMETERS 

Four parameters are used to characterize spatial properties, density of summits, texture aspect ratio, directionality of surface lay and Fastest decay autocorrelation length.

(1). Density of Summits of the Surface Sds
This is the number of summits of a unit sampling area, which relies on the eight nearest neighbour summit definition. I.e. a peak is defined of it is higher than its 8 nearest neighboures.

                                 ( 5 )

(2). Texture Aspect Ratio of the Surface Str
This is a parameter used to identify texture strength i.e. uniformity of texture aspect.  It is defined by Areal autocorrelation function (AACF). Str can be defined as its ratio of the fastest to slower decay to correlation length, 0.2, of the AACF function.
 

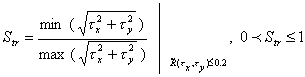     ( 6 )

where 

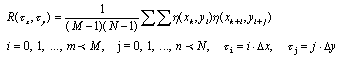
In principle, the texture aspect ratio has a value between 0 and 1.  Larger values, say Str>0.5, of the ratio indicates uniform texture in all directions i.e. no defined lay, Smaller values, say Str<0.3, indicates an increasingly strong directional  structure or lay.  Since the size of the sampling area is finite, it is possible that the slowest decay of the AACFs of some anisotropic surfaces never reaches 0.2 within the sampling area. In this case the longest distance of the AACF along the slowest decay direction can be used instead. 

(3). The Fastest Decay Autocorrelation Length Sal
This is a parameter in length dimension used to describe the autocorrelation character of the AACF.  It is defined as the horizontal distance of the AACF which has the fastest decay to 0.2. In other words the Sal is the shortest autocorrelation length that the AACF decays to 0.2 in any possible direction.

                     ( 7 )

For an anisotropic surface Sal is in a direction perpendicular to the surface lay. A large value of Sal denotes that the surface is dominated by low frequency (or long wavelength) components. While a small value of the Sal denotes the opposite situation.

(4). Texture Direction of the Surface Std
This is the parameter used to determine the most pronounced direction of the surface texture with respect to the y axes within the frequency domain, i.e. it gives the lay direction of the surface.  A unified definition of the texture direction of a surface is given an angle.  By this definition, when the measurement trace direction is perpendicular to the lay (this is a very common case) the texture direction is  .

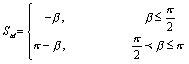               ( 8 )

where,  is the position where the maximum value of the angular spectrum.

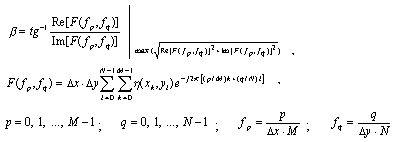
HYBRID PARAMETERS

The hybrid property is a combination of both amplitude and spacing.  Any changes that occur in either amplitude or spacing may have an effect on the hybrid property.  Three hybrid parameters are calculated here. 

(1). Root-Mean-Square Slope of the Surface 
This is the root-mean-square value of the surface slope within the sampling area.

                              ( 9 )

Where 

 

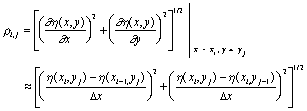
(2). Arithmetic Mean Summit Curvature of the Surface Ssc
This is defined as the average of the principal curvatures of the summits within the sampling area. Since the sum of the curvatures of a surface at a point along any two orthogonal directions is equal to the sum of the principal curvatures.
 

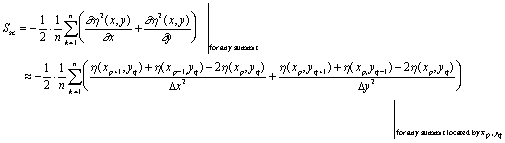        ( 10 )

This parameter can only be calculated after the summits.
 

(3). Developed Interfacial Area Ratio Sdr
This is the ratio of the increment of the interfacial area of a surface over the sampling area.

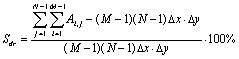                                  ( 11 )

Where the interfacial area of the quadrilateral is

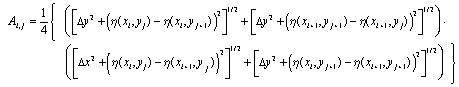
The developed interfacial area ratio reflects the hybrid property of surfaces. A large value of the parameter indicates the significance of either the amplitude or the spacing or both.
 

FUNCTIONAL PARAMETERS

INDEX FAMILY
The functional indexes proposed here are applicable to all kinds of typical engineering surfaces (highly stressed or ordinary).

(1) Surface Bearing Index Sbi
This is the ratio of the RMS deviation over the surface height at 5% bearing area.

                                                                 ( 12 )
 

where  is normalised the surface height at 5% bearing area.  A larger surface bearing index indicates a good bearing property. 

(2). Core Fluid Retention Index Sci
This is the ratio of the void volume of the unit sampling area at the core zone (5% ~ 80% bearing area) over the RMS deviation.

            ( 13 )

A larger Sci indicates a good fluid retention.  For a Gaussian surface, this index is about 1.56. 

(3). Valley Fluid Retention Index Svi
This is the ratio of the void volume of the unit sampling area at the valley zone (80% ~ 100%) over the RMS deviation.

                 ( 14 )

Where 

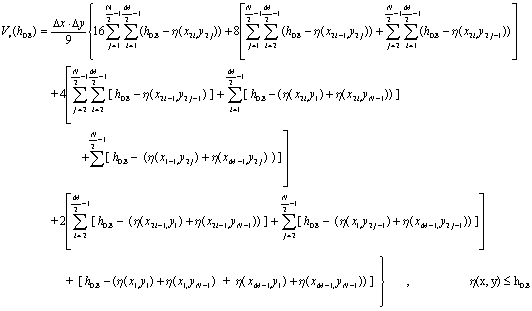
A larger Svi indicates a good fluid retention in the valley zone. 
 

VOLUME FAMILY
The material volume and void volume in the surface bearing area is a naturally geometrical descriptor of a surface topography.  The material volume and void volume enclosed in the contacting surface of the material, they may have a very close relation with functional properties of the surface, such as bearing, wear, running in and fluid retention. Three volume parameters, are presented here to characterize the functional properties of surfaces.

(1). Materiel Volume of the Surface Sm
Firstly, the material volume is defined as the material portion enclosed in the 10% bearing area and  normalised to unity.

                            ( 15 )

where 

 

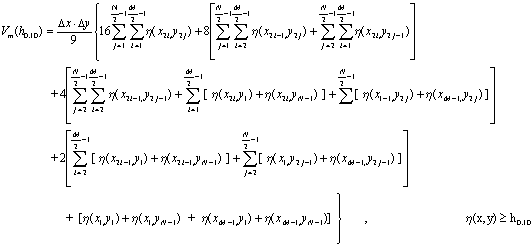
The material volume and the material volume ratio are not only geometrical descriptors of the surface, but also have significant functional implications. The material volume may reflect wear and the running-in properties. On the other hand, for a "flat-topped" surface, such as a honed surface, the material volume ratio may increase quickly, whereas for a spiked surface, such as a bored surface, the function shows a slow increase with the truncation level. Thus functionally, the material volume reflects the resistance against wear and friction. Surfaces with a rapid increase in the material volume ratio show good running-in properties whereas those with a slow increase of the functions indicates that the top part of the material is easily worn. 

(2). Core Void Volume of the Surface Sc
A core void volume is enclosed from10% to 80% of surface bearing area and normalised to the unit sampling area. 

                                        ( 16 )

(3). Valley Void Volume of the Surface Sv
The valley void volume of the unit sampling area is defined as a void volume at the valley zone from 80% to 100% surface bearing area.

                                     ( 17 )

The void volumes is proposed here to provide a direct inspection of lubrication and fluid retention of surfaces.  It represents the fluid retention ability of a highly wear surface.  For a flat topped surface, such as a honed surface, the core void volume may decrease quickly with the truncation level, whereas for a spiked surface, such as a bored surface, the function shows a slow decrease. Thus functionally, the void volumes reflect the fluid retention property.